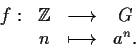![\begin{displaymath}\begin{array}{cccc}
\psi: & G/\ker f & \longrightarrow & Imf\\
& [x] & \longmapsto & f(x)
\end{array} \end{displaymath}](img4.gif)
è ben definita, ed è un isomorfismo di gruppi.
Dimostrazione
Poniamo ![]()
![]() è ben definita:
è ben definita:
se ![]() ,
,
![]() ,
cioè
,
cioè
![]() ,
per un certo
,
per un certo ![]() ,
e quindi
,
e quindi
![]() .
Allora
.
Allora
![]()
![]() è un omomorfismo:
è un omomorfismo:
![]() ,
,
![]()
![]() è biunivoca:
è biunivoca:
![]() è suriettiva in quanto
è suriettiva in quanto
![]() ,
,
![]() tale che
tale che
![]() ,
ed è iniettiva essendo
,
ed è iniettiva essendo
![]()