equazioni parametriche

23 OSSERVAZIONE-DEFINIZIONE Equazioni parametriche di un sottospazio proiettivo
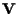
Poiché ogni sottospazio vettoriale di $\mathbf{V}$ possiede una base, ogni sottospazio proiettivo

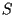
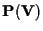
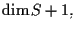
di $\mathbf{P(V)}$ può essere generato da un numero finito, pari a $\dim S+1,$ di suoi punti linearmente indipendenti.
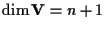
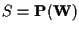
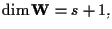
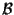
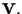
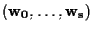
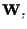
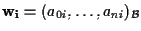
Supponiamo per esempio che $\dim \mathbf{V}=n+1$ e che $S=\mathbf{P(W)}$ con $\dim \mathbf{W}=s+1,$ e consideriamo una base $\mathcal{B}$ di $\mathbf{V}.$ Sia inoltre $(\mathbf{w_0},\ldots,\mathbf{w_s})$ una base di $\mathbf{W},$ con $\mathbf{w_i}=(a_{0i},\ldots,a_{ni})_{\mathcal{B}}$ per $i=0,\ldots,s.$
Allora abbiamo un sistema di

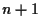
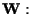
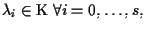
equazioni parametriche del sottospazio $\mathbf{W}:$ $(\ast) \left\{ \begin{array}{l} x_0=\lambda_0 a_{00}+\cdots + \lambda_s a_{0s} \\ \vdots \\ x_n=\lambda_0 a_{n0}+\cdots + \lambda_s a_{ns} \end{array} \right.$ con $\lambda_i \in \mathrm{K} \; \forall i=0,\ldots,s,$ e $\mathrm{r} \left( \begin{array}{ccc} a_{00} & \ldots & a_{0s} \\ \vdots & \ddots & \vdots \\ a_{n0} & \ldots & a_{ns} \end{array} \right)=s+1.$
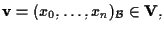
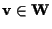
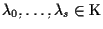
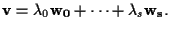
Infatti, dato $\mathbf{v}=(x_0, \ldots, x_n)_{\mathcal{B}} \in \mathbf{V},$ allora $\mathbf{v} \in \mathbf{W}$ se e solo se esistono $\lambda_0,\ldots,\lambda_s \in \mathrm{K}$ tali che $\mathbf{v}=\lambda_0 \mathbf{w_0} +\cdots +\lambda_s \mathbf{w_s}.$
Osserviamo che le equazioni $(\ast)$ si possono pensare come equazioni parametriche di

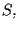
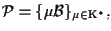
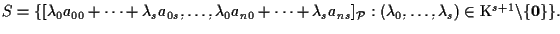
infatti $S=\mathbf{P(W)}=\{ [\mathbf{v}]:\mathbf{v} \in \mathbf{W \backslash \{0\}} \},$ cioè, posto $\mathcal{P} = \{ \mu \mathcal{B} \}_{\mu \in \mathrm{K}^{\ast}},$ $S=\{ [\lambda_0 a_{00}+\cdots + \lambda_s a_{0s}, \ldots, \lambda_0 a_{n0}+\cd... ...ambda_0, \ldots, \lambda_s) \in \mathrm{K}^{s+1} \backslash \{\mathbf{0}\} \}.$
Si noti che $S=L([\mathbf{w_0}],\ldots,[\mathbf{w_s}])$ (esempio 17 della sezione "Sottospazi proiettivi").
Si osservi poi che le $(\ast)$ dipendono dalla scelta degli

punti $[\mathbf{w_0}],\ldots,[\mathbf{w_s}]$ di

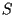
e dalla scelta dei rappresentanti $\mathbf{w_0},\ldots,\mathbf{w_s}$ di tali punti.
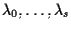
Inoltre, se attribuiamo a $\lambda_0, \ldots, \lambda_s$ i valori della

-pla $(t_0,\ldots,t_s)$ e della

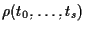
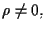
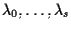
-pla $\rho (t_0,\ldots,t_s)$ con $\rho \neq 0,$ otteniamo lo stesso punto. In piú bisogna assumere $(\lambda_0,\ldots,\lambda_s) \neq (0,\ldots,0).$ Pertanto $\lambda_0, \ldots, \lambda_s$ sono detti parametri omogenei, e si può scrivere $S=\{ [\lambda_0 a_{00}+\cdots + \lambda_s a_{0s}, \ldots, \lambda_0 a_{n0}+\cd... ...{\mathcal{P}} : [\lambda_0,\ldots,\lambda_s] \in \mathbf{P^s} (\mathrm{K}) \}.$
Quindi:
per un sottospazio proiettivo di dimensione

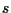
si possono trovare equazioni parametriche dipendenti da

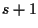
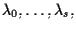
parametri omogenei $\lambda_0, \ldots, \lambda_s,$ utilizzando

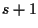
punti linearmente indipendenti del sottospazio (che è quindi generato da tali punti).
Viceversa: date equazioni del tipo $(\ast)$ con $\mathrm{r} \left( \begin{array}{ccc} a_{00} & \cdots & a_{n0} \\ \vdots & \ddots & \vdots \\ a_{0s} & \cdots & a_{ns} \end{array} \right) =s+1,$ i punti $[a_{00},\ldots,a_{n0}]_{\mathcal{P}},\ldots,[a_{0s},\ldots,a_{ns}]_{\mathcal{P} }$ sono linearmente indipendenti e generano un sottospazio proiettivo di dimensione

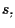
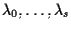
di cui le $(\ast)$ sono equazioni parametriche con $\lambda_0, \ldots, \lambda_s$ parametri omogenei.