

- 1.
- Si consideri la forma bilineare simmetrica
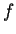 :
:
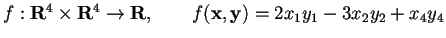
.
L'applicazione 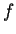 non è un prodotto scalare, infatti la sua segnatura è (2,1), quindi
non è un prodotto scalare, infatti la sua segnatura è (2,1), quindi 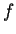 non è definita positiva, bensì indefinita.
non è definita positiva, bensì indefinita.
- 2.
- La forma
 su
su
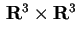 :
non è un prodotto scalare, poiché è degenere; infatti, considerando la matrice associata a
:
non è un prodotto scalare, poiché è degenere; infatti, considerando la matrice associata a 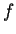 ,
si trova facilmente che
,
si trova facilmente che 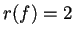 .
.
- 3.
- Si consideri la forma quadratica
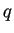 su
su
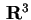 tale che
tale che
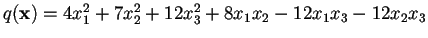
;
allora la forma bilineare simmetrica 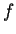 associata a
associata a 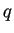 è un prodotto scalare, infatti, presa
è un prodotto scalare, infatti, presa
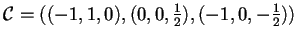 ,
base diagonalizzante per
,
base diagonalizzante per 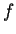 ,
si ha che:
,
si ha che:
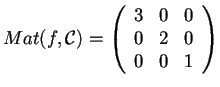
;
quindi la sua segnatura è (3,0).
Questo significa che 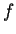 è una forma definita positiva.
è una forma definita positiva.




:
L'applicazione.
non è un prodotto scalare, infatti la sua segnatura è (2,1), quindi
non è definita positiva, bensì indefinita.
su
:
non è un prodotto scalare, poiché è degenere; infatti, considerando la matrice associata a, si trova facilmente che
.
su
tale che
allora la forma bilineare simmetrica;
associata a
è un prodotto scalare, infatti, presa
, base diagonalizzante per
, si ha che:
quindi la sua segnatura è (3,0).;
è una forma definita positiva.


 Teoria
Teoria