Esercizi

1.
Si consideri la seguente forma bilineare:
$\phi:\mathbf{R}^3 \times \mathbf{R}^3 \rightarrow \mathbf{R}, \qquad \phi(\mathbf{x},\mathbf{y})=x_{1}y_{1}+x_{2}y_{2}-x_{1}y_{3}+x_{2}y_{3}+x_{3}y_{1}$
e si determini la matrice associata di $\phi$ rispetto alla base canonica $\mathcal{E}$, a $\mathcal{B}=((1,0,0),(2,1,0),(3,0,1))$ e a $\mathcal{C}=((1,0,0),(0,0,1),(2,3,1))$.
Vuoi un aiuto?
Per vedere la soluzione totale, clicca , oppure clicca per visualizzare ogni singolo passo della soluzione.

Soluzione  
I passo:
Determinare la matrice rispetto alla base canonica è immediato, infatti basta inserire nella matrice $Mat(\phi,\mathcal{E})=(a_{ij})_{i,j=1,\ldots,3}$ il coefficiente di $x_{i}y_{j}$ nella posizione $ij$, cioè:

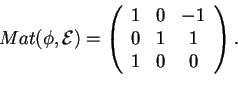
\begin{displaymath}Mat(\phi,\mathcal{E})= \begin{array}({ccc}) 1 & 0 & -1\\ 0 & 1 & 1\\ 1 & 0 & 0 \end{array}. \end{displaymath}


II passo:  
Nel secondo caso con $\mathcal{B}=(\mathbf{b}_{1},\mathbf{b}_{2},\mathbf{b}_{3})$, invece, dobbiamo svolgere i calcoli:
$a_{11}=\phi(\mathbf{b}_{1},\mathbf{b}_{1})=1, \qquad a_{21}=\phi(\mathbf{b}_{2},\mathbf{b}_{1})=2, \qquad a_{31}=\phi(\mathbf{b}_{3},\mathbf{b}_{1})=4,$
$a_{12}=\phi(\mathbf{b}_{1},\mathbf{b}_{2})=-1, \quad \, a_{22}=\phi(\mathbf{b}_{2},\mathbf{b}_{2})=5, \qquad a_{32}=\phi(\mathbf{b}_{3},\mathbf{b}_{2})=8,$
$a_{13}=\phi(\mathbf{b}_{1},\mathbf{b}_{3})=2, \qquad \,a_{23}=\phi(\mathbf{b}_{2},\mathbf{b}_{3})=5, \qquad a_{33}=\phi(\mathbf{b}_{3},\mathbf{b}_{3})=9.$ Da ciò segue che

\begin{displaymath}Mat(\phi,\mathcal{B})= \begin{array}({ccc}) 1 & 1 & 2\\ 2 & 5 & 5\\ 4 & 8 & 9 \end{array}. \end{displaymath}

III passo:  
Il terzo caso con $\mathcal{C}=(\mathbf{c}_{1},\mathbf{c}_{2},\mathbf{c}_{3})$ è analogo al precedente, infatti dobbiamo calcolare:
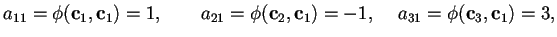
$a_{11}=\phi(\mathbf{c}_{1},\mathbf{c}_{1})=1, \qquad a_{21}=\phi(\mathbf{c}_{2},\mathbf{c}_{1})=-1, \quad \, a_{31}=\phi(\mathbf{c}_{3},\mathbf{c}_{1})=3,$
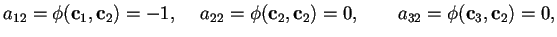
$a_{12}=\phi(\mathbf{c}_{1},\mathbf{c}_{2})=-1, \quad \, a_{22}=\phi(\mathbf{c}_{2},\mathbf{c}_{2})=0, \qquad a_{32}=\phi(\mathbf{c}_{3},\mathbf{c}_{2})=0,$
$a_{13}=\phi(\mathbf{c}_{1},\mathbf{c}_{3})=1, \qquad a_{23}=\phi(\mathbf{c}_{2},\mathbf{c}_{3})=2, \qquad a_{33}=\phi(\mathbf{c}_{3},\mathbf{c}_{3})=13.$ Da ciò segue che

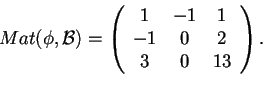
\begin{displaymath}Mat(\phi,\mathcal{B})= \begin{array}({ccc}) 1 & -1 & 1\\ -1 & 0 & 2\\ 3 & 0 & 13 \end{array}. \end{displaymath}